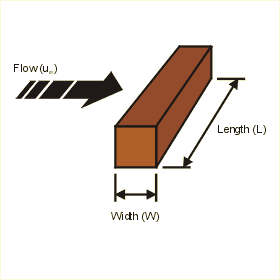
This page calculates the average heat transfer coefficient and rod temperature for an isothermal (constant temperature) circular rod in free stream flow. The convection calculation automatically switches between laminar and turbulent convection correlations based on Reynolds number.
The calculation is based on Nusselt number correlations.
The heat flow (q) from the plate is calculated as:
q = h A x (Tp - Ta)
Where h is the average heat transfer coefficient, A is the area of the rod in cross flow (W x L x 4), Tr is the rod temperature and Ta is the ambient fluid temperature. h is defined as:
h = Nu k / W
Where Nu is the Nusselt Number, k the conductivity of the fluid and W the width of the rod. The Nusselt number is calculated as:
Nu = C Ren Pr0.33
Where Re is the Reynolds number, Pr is the Prandtl number, and C and n are 0.102 and 0.675 respectively.
The Reynolds number and Prandlt number are calculated using fluid properties as follows:
Pr = kinematic viscosity / thermal diffusivity
Re = fluid velocity x width / kinematic viscosity
In addition, you must define the fluid properties at the film temperature Tf defined as follows:
Tf = (Tr + Ta) / 2
The above correlations are valid for Reynolds numbers in the range of 5000 and 100000 and Prandtl numbers in the range of 0.6 and 50. These calculations are not suitable for low Prandtl fluids like liquid metals and high Prandtl fluids like heavy oils or silicons.
References
Holman, J.P., Heat Transfer, 7th ed., McGraw Hill Book Company, New York, 1990. p 281 - 306